You Can't Always Hear the Shape of a Drum
In 1966, Marc Kac posed the question "Can you hear the shape of a drum?"More precisely, can you deduce the shape of a plane region by knowing the frequencies at which it resonates (where, as in a physical drum, the boundary is assumed to be held fixed)?
Long before Kac posed this question, mathematicians had been investigating the analogous questions in higher dimensions: Is a Riemannian manifold (possibly with boundary) determined by its spectrum?
The problem was first settled, in the negative, in higher dimensions. In 1964, John Milnor found two distinct 16-dimensional manifolds with the same spectrum. But the problem for plane regions remained open until 1991, when Carolyn Gordon, David Webb, and Scott Wolpert found examples of distinct plane "drums"which "sound" the same. See the illustrations below.
For more on the problem and its solution see "One cannot hear the shape of a drum," by Carolyn Gordon, David L. Webb and Scott Wolpert, Bulletin of the AMS, 27 (1992).
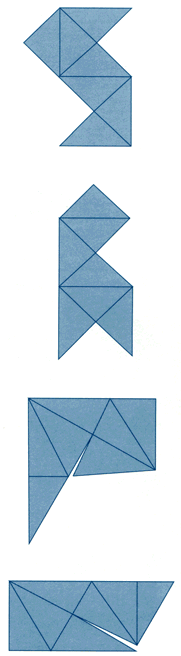  |
 David Webb and Carolyn Gordon, former faculty at Washington David Webb and Carolyn Gordon, former faculty at Washington
University in St. Louis, with paper models of a pair of
"sound-alike" drums.
(Photo courtesy of Washington University Photographic Services).
View an animation of the top two drums in Figure 1 beating.
(Reproduced with permission of the Cornell TheoryCenter)
(Note: This animation is a large file (1.7 megabytes). It is in MPEG format, so you musthave an MPEG player to view this file. If you need to locate aplayer, here is a list of some of the MPEG resourceson the web.)
|
- Steven Weintraub
Welcome to the
Feature Column!
These web essays are designed for those who have already discovered the joys of mathematics as well as for those who may be uncomfortable with mathematics.
Read more . . .
Feature Column at a glance




 David Webb and Carolyn Gordon, former faculty at Washington
David Webb and Carolyn Gordon, former faculty at Washington