The Early History of Calculus Problems, II

Some "intriguing facts" from the 18th century using fluxions. ...

Tony Phillips
Stony Brook University
Email Tony Phillips

Introduction

The first installment of this set traced back standard first-year calculus maximum-minimum problems to what may be their first occurrence in print, worked-out examples in the Marquis de l'Hospital's 1696 Analyse des infiniment petits, pour l'intelligence des lignes courbes. As the Marquis explained, he did not cover integral calculus so as not to overlap with Leibnitz's forthcoming De Scientiâ infiniti, which, Leibnitz had told him, "must include all the most intriguing facts about the inverse tangent Method, about rectifications of curves, about quadrature of the spaces they enclose, about those of the surfaces of the bodies they describe, about the dimensions of those bodies, about the location of centers of gravity, &c." Unfortunately De Scientiâ infiniti, if written, was never published.

Some of those "intriguing facts" can be found in The Doctrine and Application of Fluxions (London, 1750), by Thomas Simpson FRS; it contains "The Solution of a Variety of New, and Very Interesting, Problems in different Branches of the MATHEMATICKS." Simpson (1710-1761, best known today for "Simpson's Rule") worked in the Newtonian tradition of fluxions (differentials) and fluents (indefinite integrals) with the $\dot{x}$ notation for the differential $dx$; once this is understood the translation to current language is easy. I have transcribed the original text literally including $p$ for $\pi$ and the use of $\overline{\mbox{vinculum}}$ for (parentheses), except that I have shortened all the long eʃʃes. Images are from a copy of the second edition (1776) in the Internet Archive.

Section VII. Of the Use of Fluxions in finding the Areas of Curves.

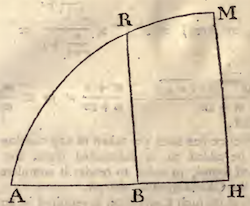parabola EXAMPLE II. Let the Curve ARMH, whose Area you would find, be the common Parabola.

In which Case the Relation of AB ($x$) and BR ($y$) being expressed by $y^2=ax$ (where $a$ is the Parameter) we thence get $y=a^{\frac{1}{2}} x^{\frac{1}{2}}$; and therefore $\dot{u}~ (=y\dot{x}) = a^{\frac{1}{2}} x^{\frac{1}{2}}\dot{x}~$: Whence $u = \frac{2}{3}\times a^{\frac{1}{2}} x^{\frac{3}{2}} = \frac{2}{3} a^{\frac{1}{2}} x^{\frac{1}{2}}\times x = \frac{2}{3}yx$ (because $a^{\frac{1}{2}} x^{\frac{1}{2}} = y$) $= \frac{2}{3}\times$ AB$\times$ BR: Hence a Parabola is $\frac{2}{3}$ of a Rectangle of the same Base and Altitude.
  • In more modern notation, $u(x)$ is the area under the curve between A and $x$; Simpson has explained that in a case like this $du = y~ dx$, i.e. $u = \int y~ dx$.

 

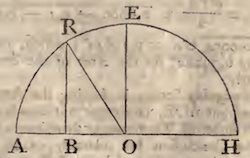semi-circle EXAMPLE IX. Let it be proposed to find the Area of a Semi-circle AREH.

 

Here, putting the Diameter AH $=a$, AB $=x$, and BR $=y~$ &c. (as usual) we have $y^2$ (BR$^2$) $=ax-xx$ (AB$\times$BH), and consequently $\dot{u}$ ($y\dot{x}$) $=\dot{x}\sqrt{ax-xx}$ $=\displaystyle{ a^{\frac{1}{2}} x^{\frac{1}{2}} \dot{x}\times \overline{1-\frac{x}{a}}^{\frac{1}{2}}}:$ Which Expression not being of the Kind [described previously], that admit of Fluents in finite Terms, let it be therefore resolved into an Infinite Series and you will have

$\displaystyle{\dot{u} = a^{\frac{1}{2}} x^{\frac{1}{2}} \dot{x}\times \overline{1-\frac{x}{2a}-\frac{x^2}{8a^2}-\frac{x^3}{16a^3} -\frac{5x^4}{128a^4}}}~$ &c.
$\displaystyle{ =a^{\frac{1}{2}}\times \overline{ x^{\frac{1}{2}}\dot{x} -\frac{x^{\frac{3}{2}}}{2a}\dot{x} -\frac{x^{\frac{5}{2}}}{8a^2}\dot{x} -\frac{x^{\frac{7}{2}}}{16a^3}\dot{x}}}~$ &c. From whence, the Fluent of every Term being taken, according to the common Method, there will come out $u =\displaystyle{=a^{\frac{1}{2}}\times \overline{ \frac{2x^{\frac{3}{2}}}{3} -\frac{x^{\frac{5}{2}}}{5a} -\frac{x^{\frac{7}{2}}}{28a^2} -\frac{x^{\frac{9}{2}}}{72a^3} -\frac{5x^{\frac{11}{2}}}{704a^4}}}~$&c. $=\displaystyle{x\sqrt{ax}\times \overline{ \frac{2}{3} -\frac{x}{5a} -\frac{x^2}{28a^2} -\frac{x^3}{72a^3} -\frac{5x^4}{704a^4}}}~$&c. = the Area ABR. Now, when $x=\frac{1}{2}a$, the Ordinate BR will coincide with the Radius OE; in which Case the Area becomes $=\frac{1}{2}a\sqrt{\frac{1}{2}aa}\times \overline{ \frac{2}{3} -\frac{1}{10} -\frac{1}{112} -\frac{1}{576} -\frac{5}{11264} }~$&c. $=\displaystyle{\frac{a^2\sqrt{\frac{1}{2}}}{2}\times \overline{0,6666-0,1-0,0089-0,0017-0,0004 }}~$&c. $=0,1964a^2$; which, when multiplied by 2, gives $0,3928a^2$ for the Area of the Semi-circle AEH, nearly.
  • This example shows first that the trigonometric substitution $x=\sin\theta$, which is now part of the ABC of integral calculus, was not in use in England in 1750. It also gives an explicit example of the use of infinite series in calculus via Newton's Binomial Theorem, for which Simpson provides an elegant proof on p. 110. The accuracy here corresponds to $\pi=3.1424$. In the rest of this example, Simpson shows how with $x=a/4$ the first four terms in the series, plus the area of the triangle OBR ($=a\sqrt{3}/4$), the whole multiplied by 3, gives a value 0.7853982 for $\pi/4$ "true to the last Decimal Place."

Comments?

SECTION VIII. The Use of Fluxions in the Rectification, or finding the Lengths, of Curves.

EXAMPLE I. Let the Curve ARM whose length is sought, be the Semi-cubical Parabola.
[Same image as Section VII, Example II.]

 

Whereof the Equation being $ax^2=y^3$, or $x = {\displaystyle \frac{y^{\frac{3}{2}}}{a^{\frac{1}{2}}} }$, we thence have $\dot{x}={\displaystyle \frac{3y^{\frac{1}{2}}\dot{y}}{2a^{\frac{1}{2}}} }$: Whence $\dot{z}~ (=\sqrt{\dot{y}^2 + \dot{x}^2}) = \displaystyle{\sqrt{\dot{y}^2 +\frac{9y\dot{y}^2}{4a}}} = \displaystyle{\frac{\dot{y}\times \overline{4a + 9y}^{\frac{1}{2}}}{2a^{\frac{1}{2}}}.}$ Whose Fluent (found by the common Rule) is ${\displaystyle \frac{\overline{4a + 9y}^{~\frac{3}{2}}} {27a^{\frac{1}{2}}}}$; which, corrected (by making $y=0$) becomes ${\displaystyle \frac{\overline{4a + 9y}^{\frac{3}{2}}} {27a^{\frac{1}{2}}}-\frac{8a}{27}=z}$.
  • Here we would write $z(y)$ for the length of the curve between ordinates $0$ and $y$. As established earlier in the book, $dz = \sqrt{dy^2 + dx^2}$, so $dz = \cdots = \displaystyle{\frac{(4a + 9y)^{\frac{1}{2}}}{2a^{\frac{1}{2}}}~dy}$. The indefinite integral, by substitution, is ${\displaystyle \frac{(4a + 9y)^{\frac{3}{2}}} {27a^{\frac{1}{2}}}}$ which evaluated at the endpoints $0$ and $y$ gives the answer.

SECTION IX. The Application of Fluxions in investigating the Contents of Solids.

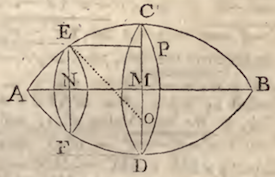spindle EXAMPLE VII. Where it is required to find the Content of a Parabolic Spindle; generated by the rotation of a given Parabola ACB about its ordinate AB.

Put CM (the Abscissa of the given Parabola) $=a$, and the Semi-ordinate AM (or BM) $=b$; and, supposing ENF to be any Section of the Solid parallel to DC, let its Distance MN (or EP) from DC, be denoted by $w$. Then, by the Property of the Curve, we shall have $\mbox{AM}^2~(b^2) : \mbox{EP}^2~(w^2)~ :: \mbox{CM}~(a) : ~\mbox{CP} ={\displaystyle \frac{aw^2}{b^2}}$: Therefore EN (= CM$-$CP) $=a -{\displaystyle \frac{aw^2}{b^2}} =$ ${\displaystyle \frac{a\times\overline{b^2-w^2}}{b^2}}$ and consequently $p \times $EN$^2 = {\displaystyle \frac{pa^2}{b^4}}\times\overline{b^4-2b^2w^2+w^4} =$ the Area of the Section EF: Which multiply'd by $(\dot{w})$, the Fluction of MN, gives ${\displaystyle\frac{pa^2}{b^4}}\times \overline{b^4\dot{w}-2b^2w^2\dot{w}+w^4\dot{w}}$ for the Fluxion of the Solidity, whose Fluent, ${\displaystyle\frac{pa^2}{b^4}}\times \overline{b^4w-\frac{2}{3}b^2w^3+\frac{1}{5}w^5}$, when $w$ becomes $b$, is ${\displaystyle (\frac{8pa^2b}{15})}$ half the Content of the Solid.
  • More specifically, set the origin at C and let the parabola be ${\displaystyle y = -\frac{a}{b^2}w^2}$. The disc EF is the cross-section at $w$ and has area $\pi \mbox{EN}^2 = \pi (\mbox{CM}-\mbox{CP})^2$ $= {\displaystyle \frac{\pi a^2}{b^4}}(b^4-2b^2w^2+w^4)$. Hence the differential of the volume is ${\displaystyle \frac{\pi a^2}{b^4}(b^4-2b^2w^2+w^4)~dw},$ with indefinite integral ${\displaystyle \frac{\pi a^2}{b^4}(b^4w-\frac{2}{3}b^2w^3+\frac{1}{5}w^5)}$. Integrating from $0$ to $b$ gives the answer ${\displaystyle (\frac{8\pi a^2b}{15})}$, half the total volume.

 

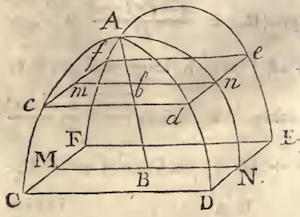
groin

EXAMPLE X. Let the proposed Solid be that, commonly know by the Name of a Groin; whose sections parallel to the Base are, all, Squares, and whereof two sections perpendicular to the base, through the Middle of the opposite Sides, are Semi-circles.

Let $bcde\!f$ be any Section parallel to the Base; and let its Distance A$b$ from the Vertex of the Solid be denoted by $x$; also let $a$ represent the Radius AB (or BN) of the circular section ABNA, perpendicular to the Base. Then, $bn$ being (by the Property of the Circle) $=\sqrt{2ax-xx}$, the Side of the square $d\!f$ will be $=2\sqrt{2ax-xx}$, and therefore the Area $=4\times\overline{2ax-xx}$; whence $\dot{s} = 4\dot{x}\times\overline{2ax-xx}$, and consequently $s=4ax^2 -\displaystyle{\frac{4x^3}{3}}$: Which, when $x=a$ becomes $\displaystyle{\frac{\overline{2a}^3}{3}} =$ the Content of the whole Solid.
  • Here we would set $s(x)$ as the volume measuring down $x$ units from the vertex. Note that the figure has an error: the curve in the rear should have its vertex at A.

Comments?

Section XI. Of the Use of Fluxions in finding Centers of Gravity, of Percussion and of Oscillation of Bodies.

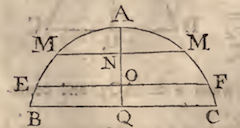section of sphere EXAMPLE IV. [Center of Gravity = O] Let ABC represent a Segment of a Sphere, or Spheroid.

 

In which Case, denoting the Axis of the Sphere, or Spheroid, by $a$, and the other Axis of the generating Curve, when an Ellipsis, by $b$, we have $y^2=\displaystyle{\frac{bb}{aa}\times\overline{ax-xx}}$; and therefore $\displaystyle{ \frac{Flu.~ y^2x\dot{x}}{Flu.~ y^2\dot{x}} = \frac{Flu.~ \overline{ax-xx}\times x\dot{x}}{Flu.~ \overline{ax-xx}\times \dot{x}} } $ $\displaystyle{=\frac {\frac{1}{3}ax^3-\frac{1}{4}x^4} {\frac{1}{2}ax^2-\frac{1}{3}x^3}= \frac{\frac{1}{3}ax-\frac{1}{4}x^2} {\frac{1}{2}a-\frac{1}{3}x}=\frac{x\times\overline{4a-3x}}{6a-4x}=}\mbox{AO}$.
  • Here the ellipsoid is ${\displaystyle \frac{X^2}{a^2} + \frac{y^2}{b^2} +\frac{z^2}{b^2} = \frac{1}{4}}$, using $a,b$ for the whole axes. Simpson's $x$ is as usual the distance from the apex: $x=a/2-X$. Also, as usual, he uses the same symbol $x$ for the height of the segment and for the variable of integration. Working in the plane $z=0$ the equation becomes $\displaystyle{\frac{(a/2-x)^2}{a^2} + \frac{y^2}{b^2} = \frac{1}{4}}$, or $\displaystyle{ y^2 = \frac{b^2}{a^2}(ax-x^2)}$. In the ellipsoid with density 1 the mass integrand is $\pi y^2~d\xi$ and the moment integrand $\pi y^2 \xi~d\xi$, using $\xi$ for the variable of integration. The coordinate of the center of gravity of the segment between 0 and $x$ is $\displaystyle{\frac{\int_0^x\pi y^2 \xi~d\xi}{\int_0^x\pi y^2 ~d\xi} = \frac{\int_0^x(a\xi-\xi^2)\xi~d\xi}{\int_0^x(a\xi-\xi^2)~d\xi} = \frac{(a\xi^3/3-\xi^4/4)|_0^x}{(a\xi^2/2-\xi^3/3)|_0^x}=\frac{x(4a-3x)}{6a-4x}}$.

     

PART II, Section IX. The Use of Fluxions in determining the Attraction of Bodies under different Forms.

[A linked set of 5 problems]
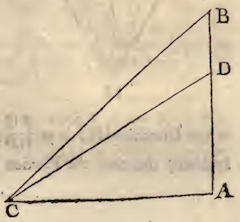PROB I. Supposing AC perpendicular to AB, and that a Corpuscle at C is attracted twards every Point or Particle of the Line AB, by Forces in the reciprocal duplicate Ratio of the Distances; to determine the Ratio of the whole Force whereby the Corpuscle is urged in the Direction CA.

 

Put $\mbox{AC} =a$, and let AD (considered as variable by the Motion of D towards B) be denoted by $x$: Then, the Force of a Particle at D being as $\displaystyle{\frac{1}{\mbox{CD}^2}}$ (by Hypothesis) its Efficacy in the proposed Direction AC will (by the Resolution of Forces) be as $\displaystyle{\frac{\mbox{AC}}{\mbox{CD}} \times \frac{1}{\mbox{CD}^2} =\frac{a}{\overline{a^2+x^2}^{\frac{3}{2}}}}$: Therefore $\displaystyle{\frac{a\dot{x}}{\overline{a^2+x^2}^{\frac{3}{2}}}}$ is the Fluxion of the whole Force; whose Fluent [as shown earlier] is $\displaystyle{\frac{x}{a\times\overline{a^2+x^2}^{\frac{1}{2}}} =\frac{\mbox{AD}}{\mbox{CA}\times\mbox{CD}}}$, will, when AD $=$ AB, be as the Force itself. Q. E. I.
  • The component of the force of the particle at D in the AC direction will be the magnitude of the force times the sine of angle CDA. i.e. times AC/CD. For the integration of $\displaystyle{\int \frac{a ~dx}{(a^2 + x^2)^{3/2}}}$ Simpson refers to an earlier section on methods of integration. Today we would use the substitution $x = a\tan\theta$ for an easier derivation. If AB = $\ell$, then the total force will be proportional to $\displaystyle {\frac{\ell}{a\sqrt{\ell^2+a^2}}}$.

 

disc

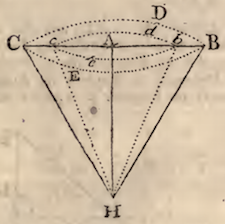
PROB. II. Supposing BCDE to represent a circular Plane, and that a corpuscle H, in the Axis thereof AH, is attracted by every Point or Particle of the Plane by Forces in the reciprocal duplicate Ratio of the Distances; to find the whole Force by which the Corpuscle is urged towards the Plane.

Let AH $=a$, and H$b=x$; then A$b^2 = x^2-a^2$; which multiply'd by $(p=3,14159$ &c.) the Area of the Circle whose Radius is Unity, gives $p\times\overline{x^2-a^2}$ for the Area of the Circle A$cdbe$: whose Fluxion is $=2px\dot{x}$. But the Force of a single Particle at $b$, in the Direction HA, is as $\displaystyle{\frac{\mbox{AH}}{\mbox{H}b^3}}$, or $\displaystyle{\frac{a}{x^3}}$ (see the last Problem) therefore the Fluxion of the whole Force is truly defined by $\displaystyle{2px\dot{x}\times\frac{a}{x^3}}$ or its equal $\displaystyle{\frac{2p\dot{x}}{x^2}}$ and the Force itself by the Fluent of $\displaystyle{\frac{2pa\dot{x}}{x^2}}$; which (properly corrected) is $\displaystyle{-\frac{2pa}{x}+ \frac{2pa}{a}} = 2p\times \overline{1-\frac{a}{x}} =2p\times \overline{1-\frac{\mbox{AH}}{\mbox{BH}}}$, when $x=$HB. Q.E.I.
  • Today it would seem more natural to work with the coordinate $r=$A$b$. Then the mass of the annulus between $r$ and $r+dr$ would be $2\pi r~dr$, the component of its attraction in the AH direction would be proportional to $\displaystyle{\frac{2\pi r~dr}{x^2}}$ times the cosine of angle AH$b$ (i.e. $\displaystyle{\frac{a}{x}}$) and the total attraction proportional to $\displaystyle{\int_0^{AB}\frac{2\pi a r~dr}{x^3} = \int_0^{AB}\!\!\!\frac{2\pi a r~dr} {(r^2+a^2)^{\frac{3}{2}}}}$, since $x=\sqrt{r^2+a^2}$. The substitution $u=r^2+a^2, du = 2r~dr$, with evaluation ("correction") at 0 and AB, leads to Simpson's answer.

[Problems III and IV apply this method to the cone and the cylinder. In each case, the attracted point is on the rotational axis of symmetry of the body, and it is understood that the components of the attraction in non-axial directions cancel out.]

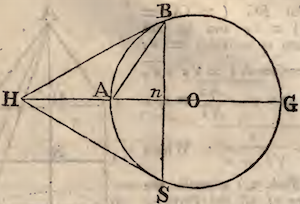sphere PROB. V. The Law of the Force being still supposed the same, to determine the attraction of a Sphere OABGS, at any given point H above its Surface.

 

Let BS be perpendicular to HG, and let HB be drawn; also put the radius AO $=a$, OH $=b$, AH $(b-a)=c$, H$n = y$ and HB $=c+x$; then A$n = y-c$, G$n=2a-y+c$, and consequently $\overline{y-c}\times \overline{2a-y+c}~ (=~$A$n~\times$ G$n =~$B$n^2 =~$BH$^2-$H$n^2) = \overline{c+x}^2 -y^2$. From which Equation we get $\displaystyle{ y=\frac{2ac+2c^2+2cx+x^2}{2a+2c}}$ (because $a+c=b$.) Whence also $\displaystyle{ 2p\times \overline{1-\frac{Hn}{HB}}= 2p\times \overline{1-\frac{2bc+2cx+x^2}{2b\times\overline{c+x}}}}$: Which multiply'd by $\displaystyle{\frac{c\dot{x}+x\dot{x}}{b}=\dot{y}}$ gives $\displaystyle{\frac{p\times\overline{2ax\dot{x}-x^2\dot{x}}}{b^2}}$ for the Fluxion of the required Force; whereof the Fluent $\displaystyle{\frac{p\times\overline{ax^2-\frac{1}{3}x^3}}{b^2}}$ will be the Attraction of the Segment ABS: Which therefore, when B coincides with G and $x$ is $=2a$, becomes $\displaystyle{\frac{4pa^3}{3b^2}}$, for the Measure of the Attraction of the whole Sphere. Q.E.I.
  • This solution is cleaner than the more straightforward modern versions (see, for example, this MIT course page). Simpson simplifies the calculation by the astute but non-intuitive use of $x=~$HB$-$HA as the variable of integration. He first uses the geometric identities $\mbox{A}n\times\mbox{G}n = \mbox{B}n \times\mbox{S}n ~(=\mbox{B}n^2)$ and $\mbox{B}n^2 = \mbox{BH}^2 - \mbox{H}n^2$ to derive the relation $\displaystyle{y= \frac{2ac+2c^2+2cx+x^2}{2(a+c)}}$ or $\displaystyle{y= \frac{2bc+2cx+x^2}{2b}}$, since $a+c=b$; and so $\displaystyle{dy = \frac{2(c+x)}{b}~dx}$. The increment $dF$ to the force, coming from the slice between $y$ and $y+dy$, is $dF = \displaystyle{2\pi(1-\frac{y}{c+x})~dy}$, using Problem II, which becomes $\displaystyle{2\pi\left (1-\frac{2bc+2cx+x^2}{2b(c+x)}\right )~\frac{2(c+x)}{b}~dx}$, which simplifies (!) to $\displaystyle{dF = \pi\frac{2ax-x^2}{b^2}~dx}$. Since $x$ varies from $0$ (when B = A) to $2a$ (when B = G), the total attraction is $\displaystyle{F=\int_0^{2a}\!\!\!dF = \frac{\pi}{b^2}(ax^2 -\frac{x^3}{3})|_0^{2a} = \frac{4\pi a^3}{3b^2}}$.
Simpson points out an important aspect of this calculation as:
COROLLARY II. Since $\displaystyle{\frac{4pa^3}{3}}$ is known to express the Content of the Sphere whose Radius is $a$, it is evident that the attraction $\displaystyle{(\frac{4pa^3}{3b^2})}$ of any Sphere is, universally, as its Quantity of Matter directly, and the Square of the Distance from its Center inversely; and is, moreover, the very same as it would be, was all the Matter in the Sphere to be united in a Point at the Center.

Comments?

Tony Phillips
Stony Brook University
Email Tony Phillips